
STAGE I: Matching rules (Alien)
A quasicrystal is formed like any other crystal, as a formation of atoms in a crystallization process. Since quasi-periodic tilings are highly ordered but don’t represent crystallographic symmetries, so that no periodic structure arises, the question is how the atoms know to organize and find their appropriate positions. The forces of directly adjacent atoms result in local growth rules, but they don’t inform the atoms to follow an overall quasicrystal pattern. To better understand the process and to maintain the global aperiodicness in the pattern, specific decorations are developed for numerable crystal units (embodying clusters of atoms) in order to describe local matching rules. They are called matching rules because the individual units are combined in such a way that their adjacent decoration match with each other. The matching rules direct the units to aggregate in a specific non-periodic order. They are still the conventional analog of the forces acting on atoms and molecules controlling the self-assembly in real crystals.
The matching rules are not perfect local growth rules. Unforced and wrong choices can cause building defects that affect the formation much later and even on an entirely different part of the aggregation. It may also be that the growth in compliance with the rules can’t be continued and the incorrect growth creates dead ends.
To avoid these dead ends, local growing can start on a special kernel. A defect in the decoration of the decapod violates the rules but specifies a pattern in which there are no dead ends to start. In general, solutions like these are not appropriate, since they don’t solve the original problem of creating a perfect formation through local growth beginning from an error-free configuration.
Matching rules as local growth rules can be assumed as the self-assembly process of natural periodic crystals, but if this is also the case for the growth of real quasicrystals is still unknown. The previously known so far are not foolproof and involve local adaptation. On the other hand, if there are absolute matching rules, the quasi-periodic tiling is determined, and their local isomorphism (LI) class is stable, which is contrary to the nature of quasicrystals.

quasicrystal cluster with matching decorations
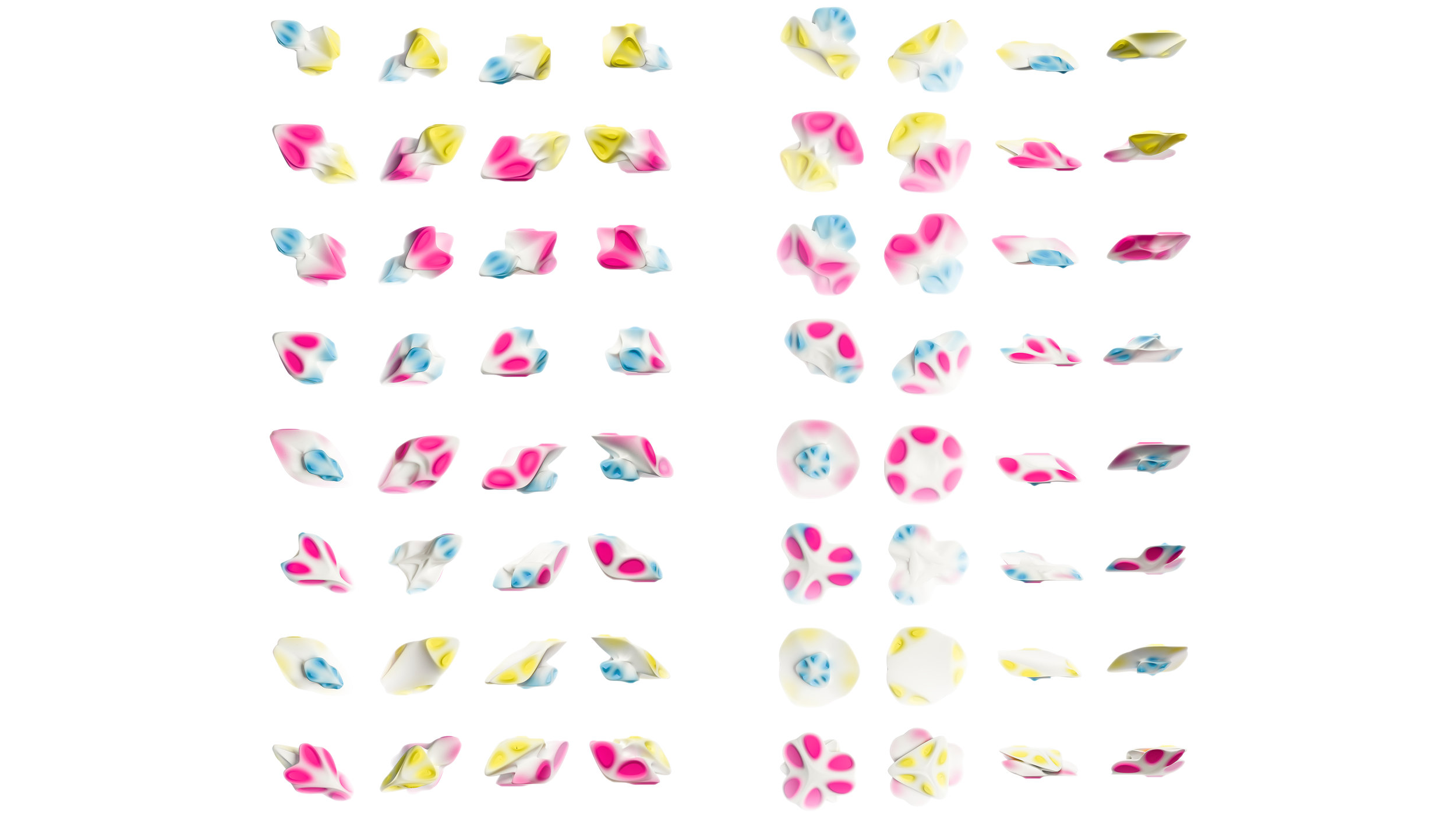
16 quasicrystal units with their specific decoration

quasicrystal and crystalquasi cluster

cyan quasicrystal formation detail

magenta quasicrystal formation detail

3D print models of quasicrystal units with magnetic decorations
