STAGE V: Algebraical pattern (Weave)
Mathematical knots describe the relation between geometry and algebra. The question of whether two given knots are topologically equivalent without “cutting” a physical “thread” is not a matter of the weaving of knots in practice, but of the mathematical properties of these weaves.
As in the generalization of crystals, the science of crystallography deals with the algebraization of different crystal formations. Crystals of different nature are not directly distinguishable, but the correlation can be determined from their mathematical origin. Like the study of various weave patterns due to their symmetry and complexity, it is also thinkable to look at these patterns by their topology of the knots. Repetitive woven textiles can be seen as two-dimensional crystals, with the base knot being periodic and infinite.
A cutout of the textile is sufficient enough to describe the pattern of the whole fabric. It represents a simple code, a sequence of up and down movements of the individual threads that recursively repeats itself over and over again. A periodic crystal defines the basic topological information of a three-dimensional weave pattern.
But here, too, the quasicrystal shows that it is literally wired differently.Each thread describes a one-dimensional quasicrystal that leads to the fact that its weaving movement is in the rhythm of a non-periodic Fibonacci series. Even though each thread follows its rhythm, it remains the aperiodic harmony with all the others. The result is a spatial quasi-crystalline weave that can always be read from different directions. It is a multi-directionalarticulation in which each thread knits its own story.
Weaves based on quasicrystals are much more differentiated and, due to their multi-directionality, enable both a regular and a more entangled nature. The simplicity of the knot itself remains, only the way the sequence of order is described gives meaning to the structure.

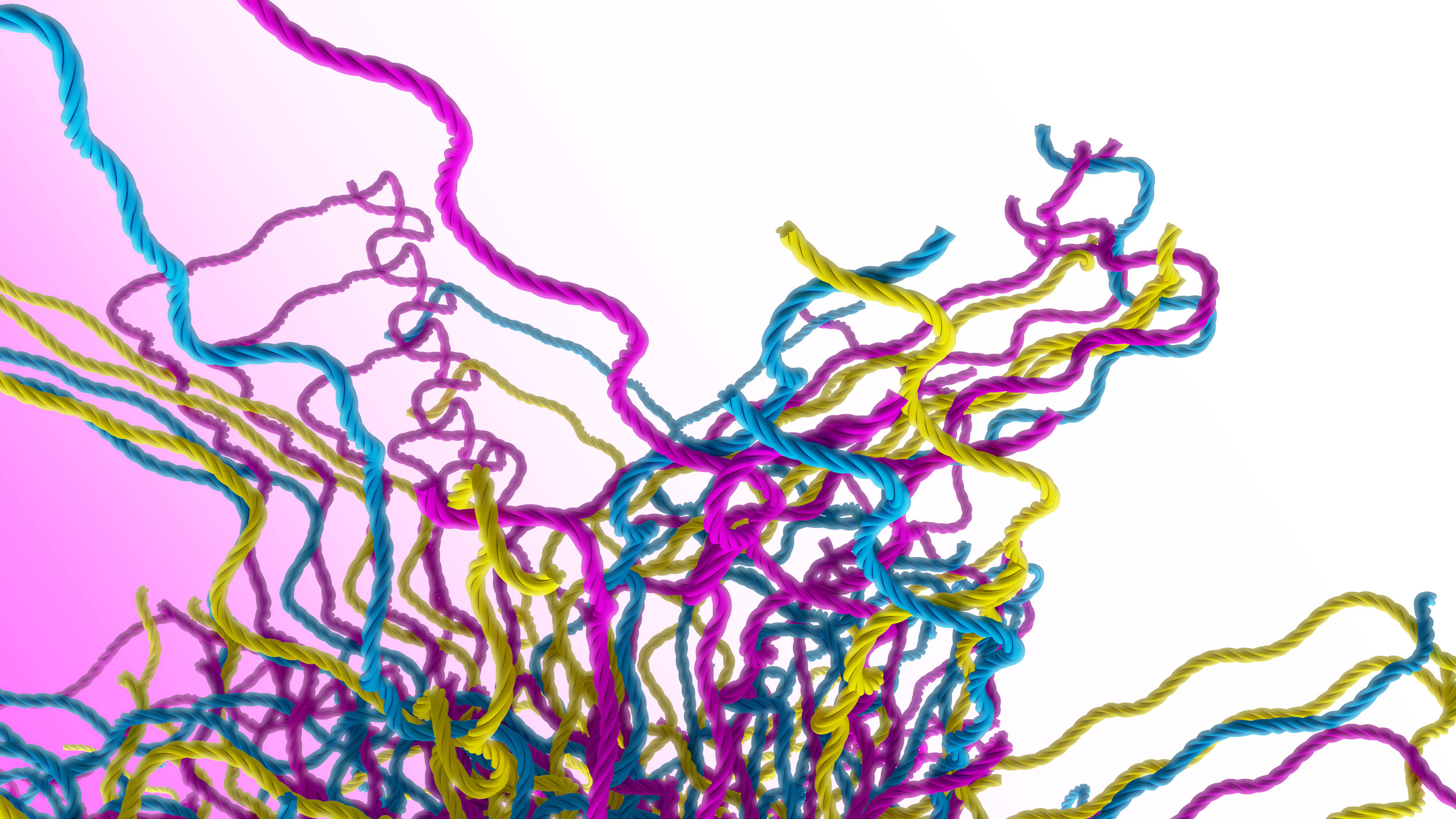
3D aperiodic thread weave with an highly entangled center

comparison regular and aperiodic 3D fabric units
entangled threads showing the high diversity of knots
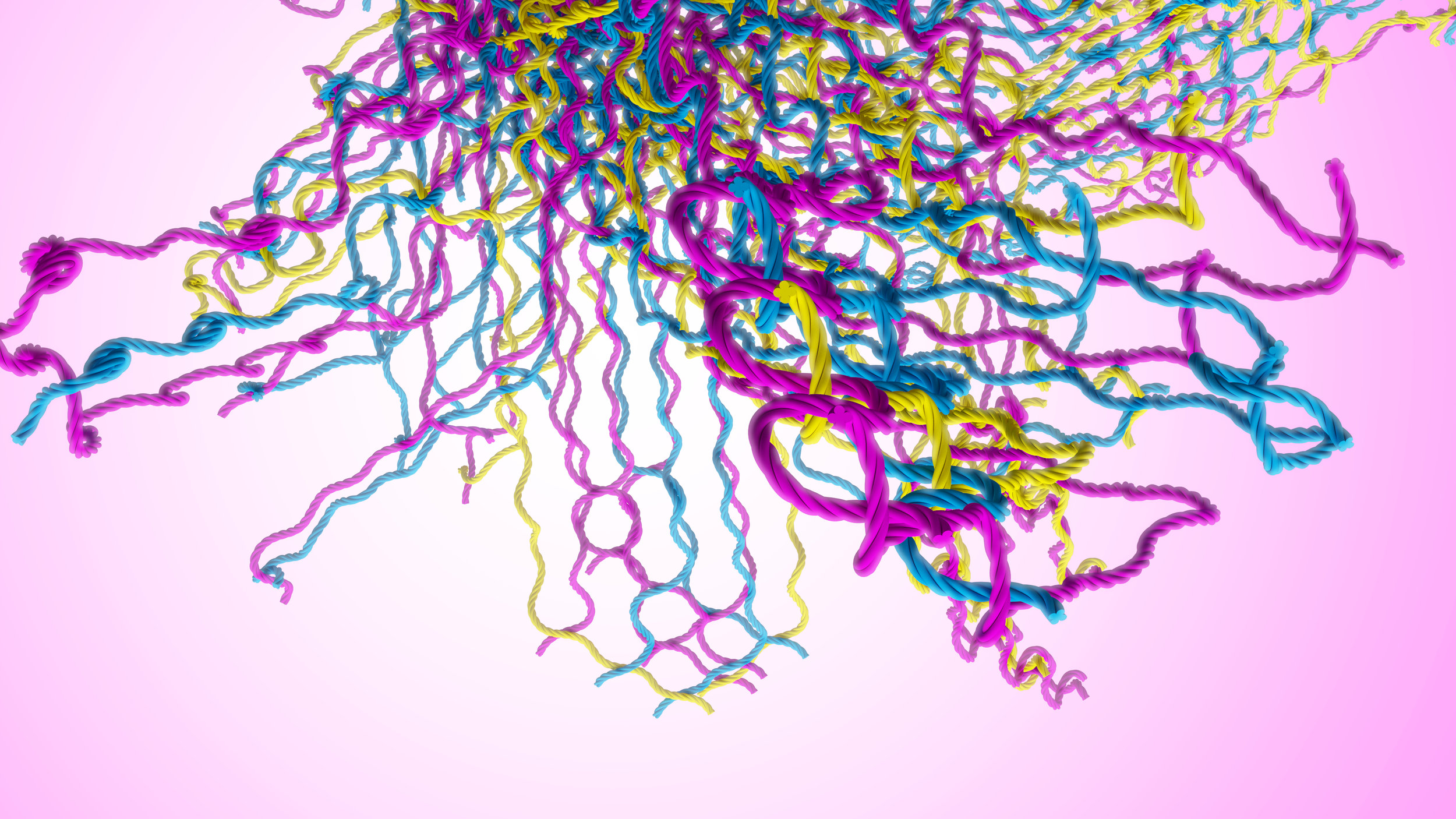
game of threads

aperiodic rhythm of threads
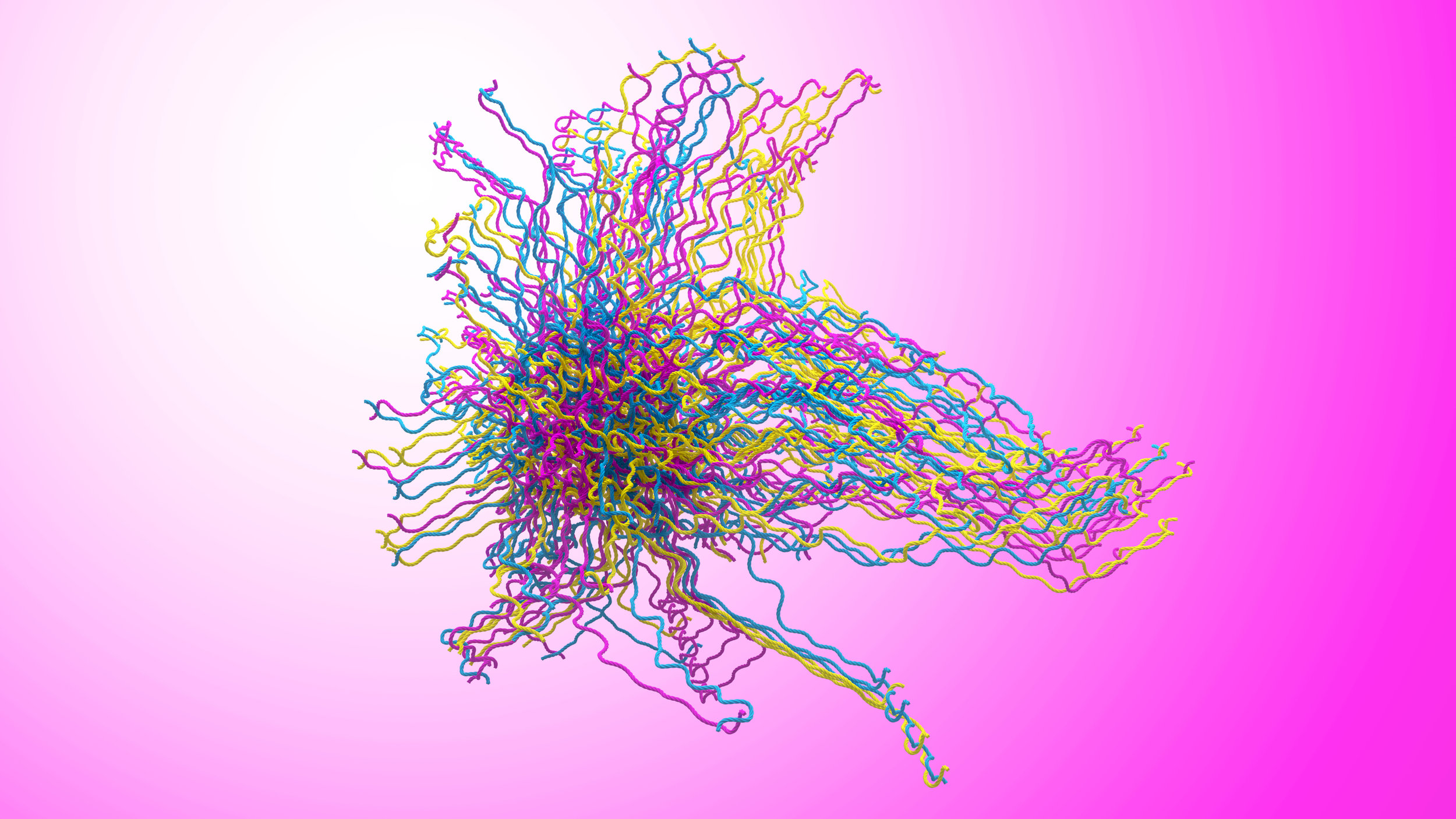
chaotic looking ball of threads
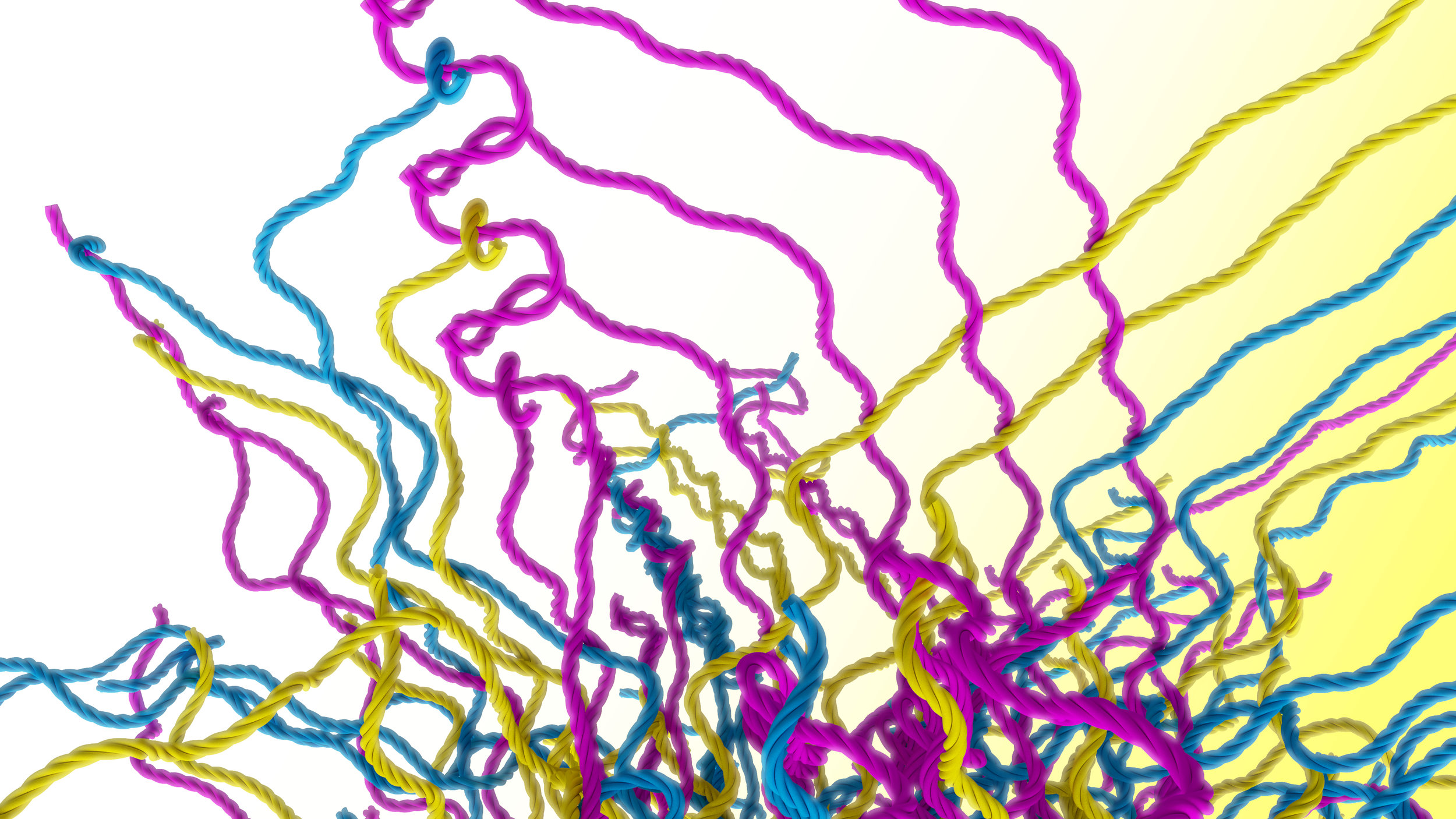
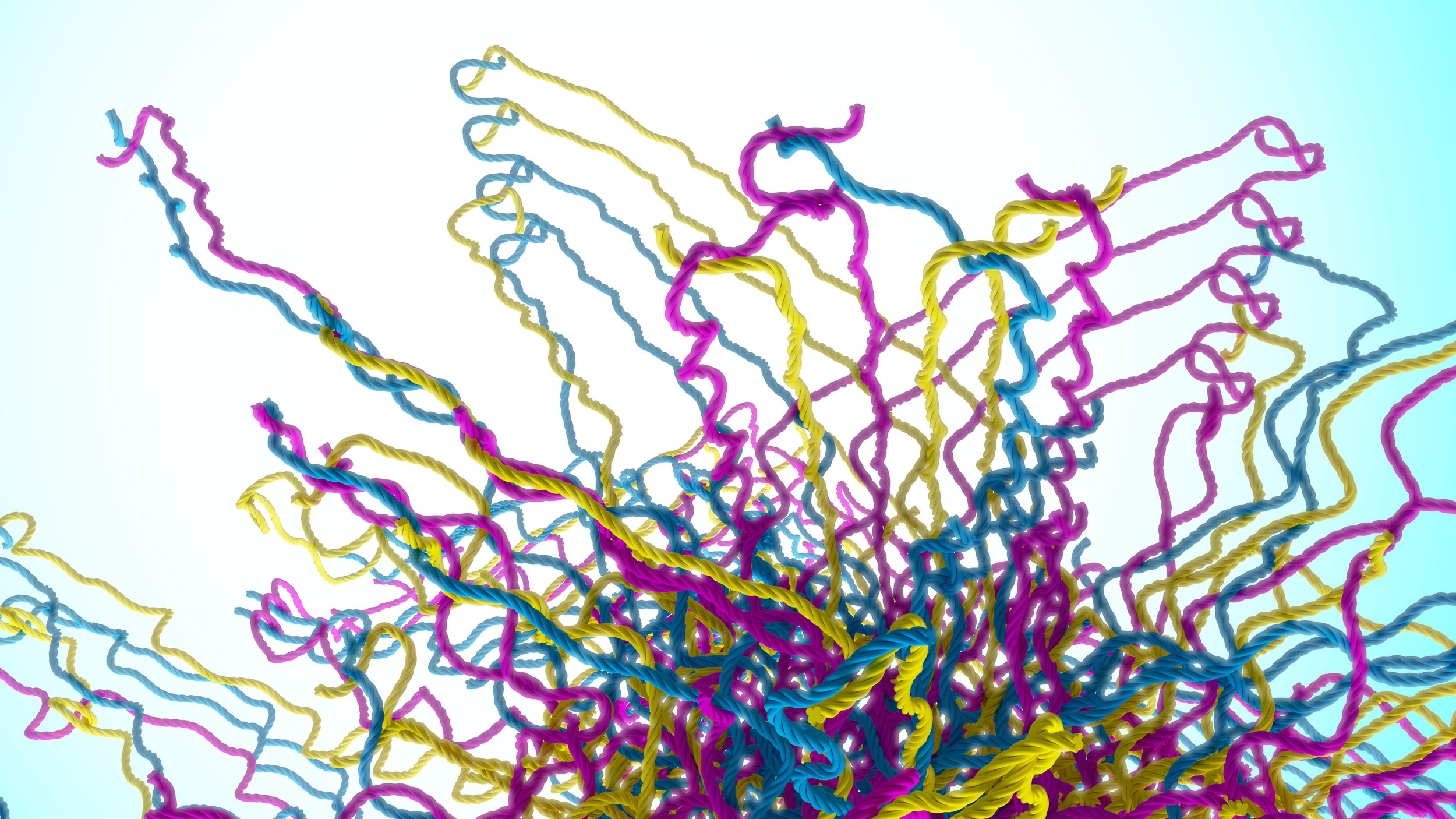
detail of the last hooks of the 3D weave