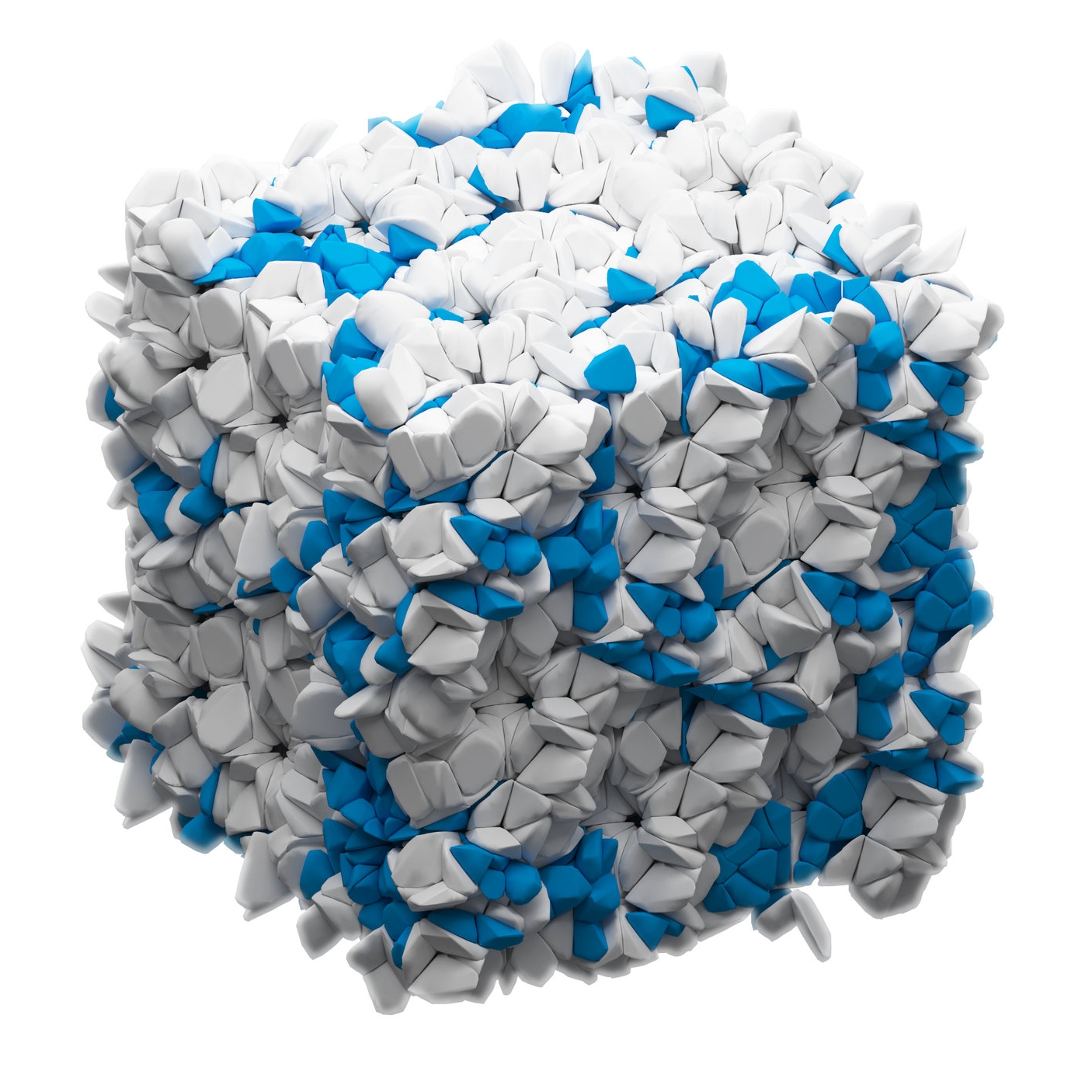
STAGE IV: Quasiperiodic aggregation (Pile)
Repetitions provide a basis for differentiation of elements within a model and can then serve for selections within several models. This involves recognizing repetitive patterns of elements or clusters in order to better understand the structure and to simplify the model in its entirety.
Thus, one can argue that in crystalline systems, which are based on continuous repetitions, the whole is not larger than the sum of the parts. The algebraic nature of crystals can describe any model by reducing it to a repetitive basis of elements with a specific extent. No matter how big the model is quantitative, the basis information remains the same. This only applies to systems in which the hierarchy of the individual elements is clearly recognizable. As these patterns become more complex, depending on the dedicated symmetry group, even a few repeating elements can create intricate structures in which the distinct parts are hard to differentiate. They need a closer look to trace back the respective symmetries. The degree of complexity increases within the information of the basis, but the global recurring pattern is still perceptible. In a crystal, the regular grid is always visible. To blur the borders of recognizing patternsin the structure, even more, the number of different elements within the system can be increased to the particular point where there are no repetitions anymore, and all parts are distinct. The result is an increase in complexity, but at the same time, the information of the whole model increases as well. Only the hierarchy gets simplified because all non-redundant elements are specific and the whole can be reduced to the individual parts. The model becomes less artificial or crystalline, as these regular formations are very rare in nature.
Quasi-crystalline arrangements dissolve this dependence of complexity decrease with repetitive elements. In a quasi-periodic arrangement, the distinction of repetitive elements within a structure is less visible and, as with non-differentiable models, still possess a high degree of complexity, but with less amount of information. The disappearance of clear recognizable patterns and, at the same time, the decrease in regularity is a suitable example, in general, for experimenting with the limits of perceptibility and differentiability. For what is described as being natural and emergent is perhaps just a certain degree of complexity that must be exceeded. Some stochastic processes are also subordinated to a hierarchy that is probably beyond our possible perception.
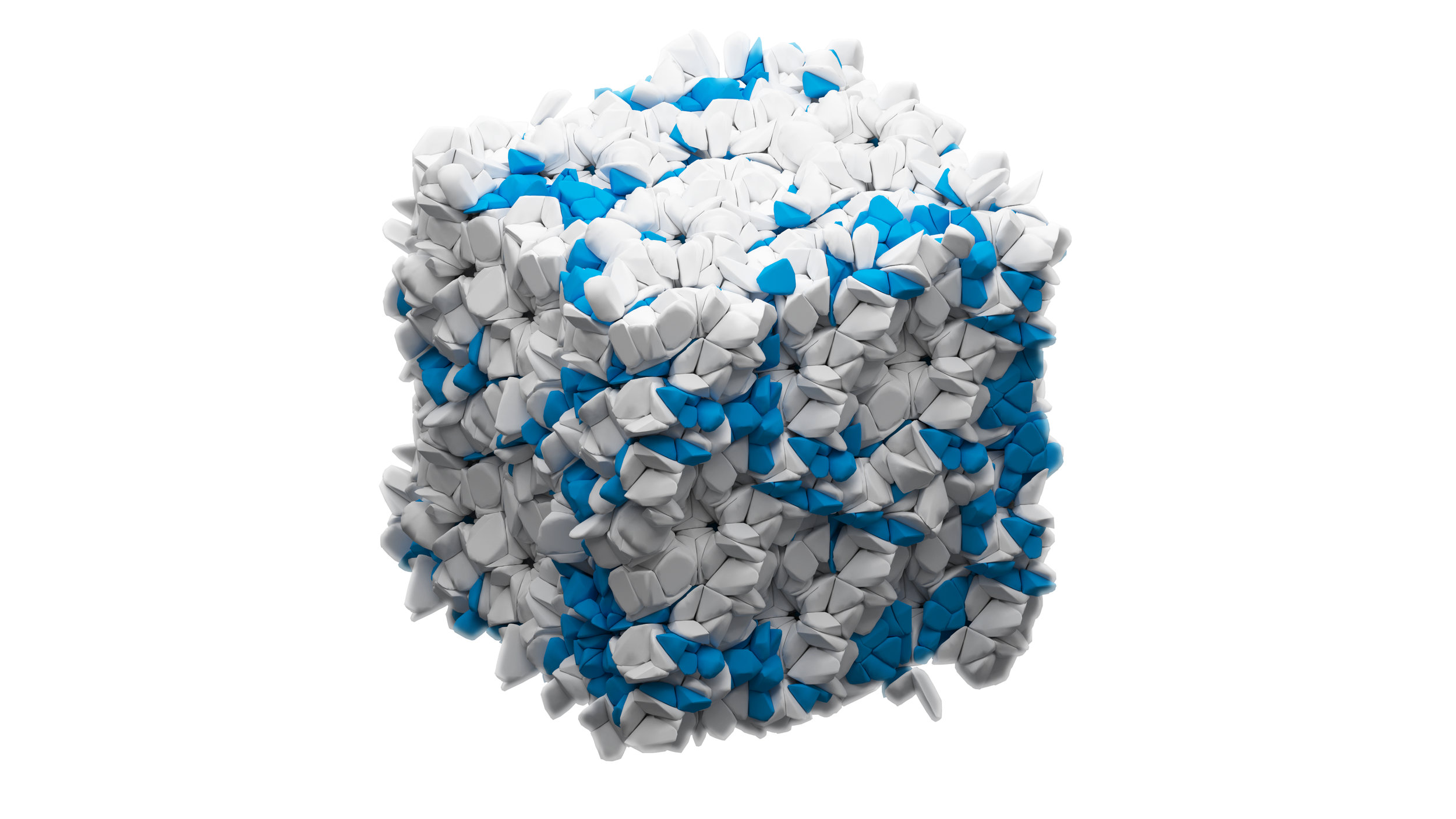
aperiodic pile of stones
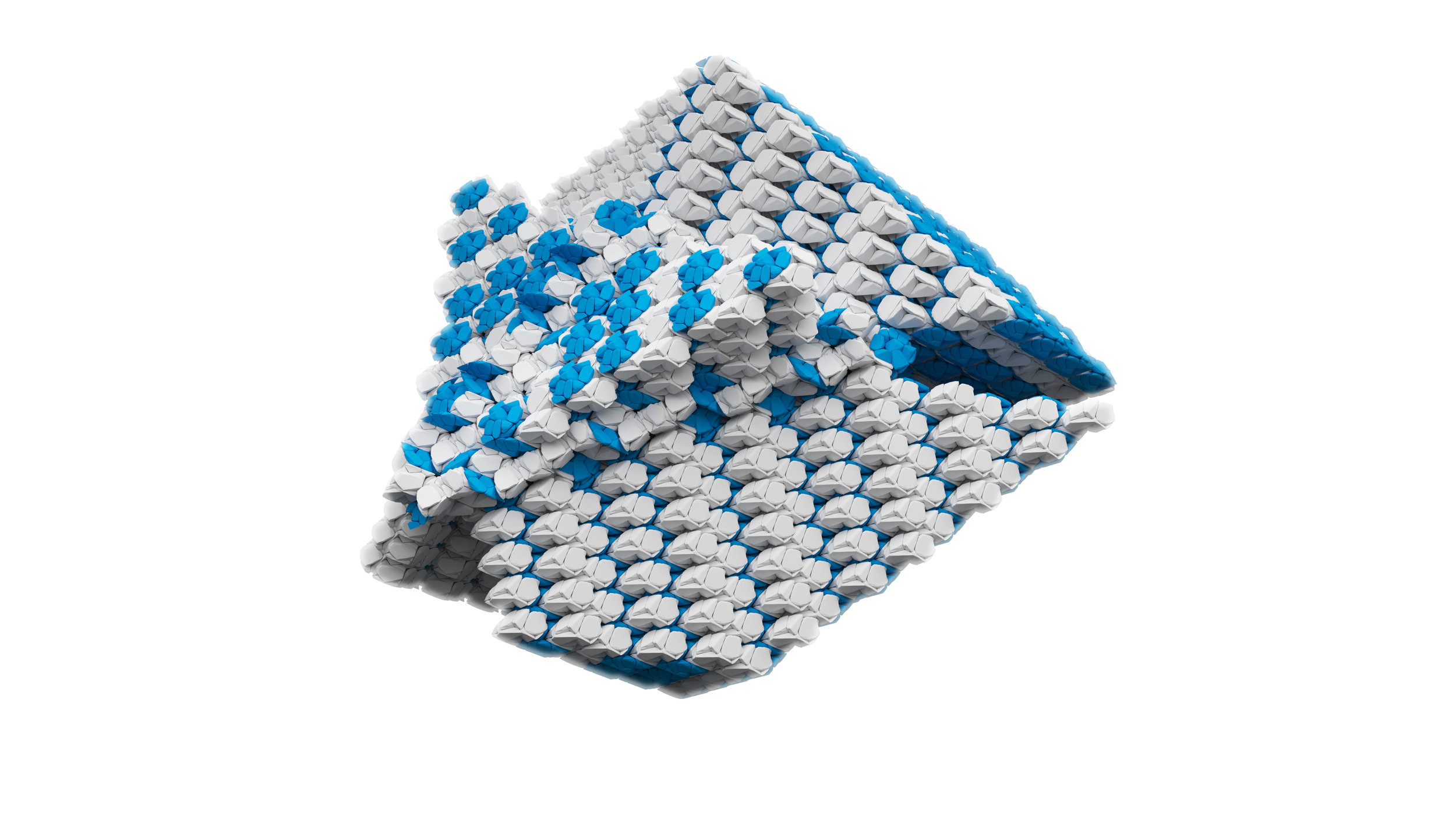
accumulation of repetitive piles of stones

comparison regular and periodic stone pile vs chaotic and aperiodic stone pile
